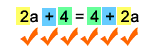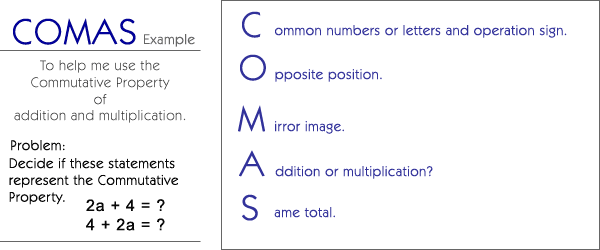
• See if each side of the equation has the same numbers and operation signs.
• Write the statements so that the first one is on the left side of the equal sign and the second one is on the right side. Use a highlighter or colored pencil to look at each side and decide if there are the same numbers and operation signs.

• Put a check mark under each highlighted number and operation sign to make sure you have the same numbers and signs in both equations.

"All the numbers and signs in both equations have check marks, that means that each equation has the same numbers and operation signs."

• Check if the numbers or letters on one side are in the opposite position on the other side.

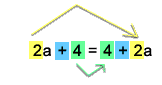
"Now I need to look at the both sides and see if the numbers on the right side of the equation are in opposite positions from the left side of the equation."

"When I look at the two equations, the "2a" is the first number on left side but is the second number on the right side. The 4 is the second number on left side but is the first number on the right side."

• The right side of the equation is the mirror image of the left side.

" When I compare 2a + 4 and 4 + 2a, they are mirror images."

A ddition or multiplication?

• The operation signs are either addition signs (+) or multiplication signs (X). Circle the operation signs in each equation.

"There is an addition sign on the left side and an addition sign on the right side."

S ame total.

• The total of each side is equal.
• Solve each equation and see if you get the same answer
  
  " If a is 2, then when I solve 2a + 4, I get the answer 8; and when I solve 4 + 2a, I get the answer 8. The total of each side is equal. This statement represents the Commutative Property."